Orifice Leakage Calculation

Saint Venant Formula

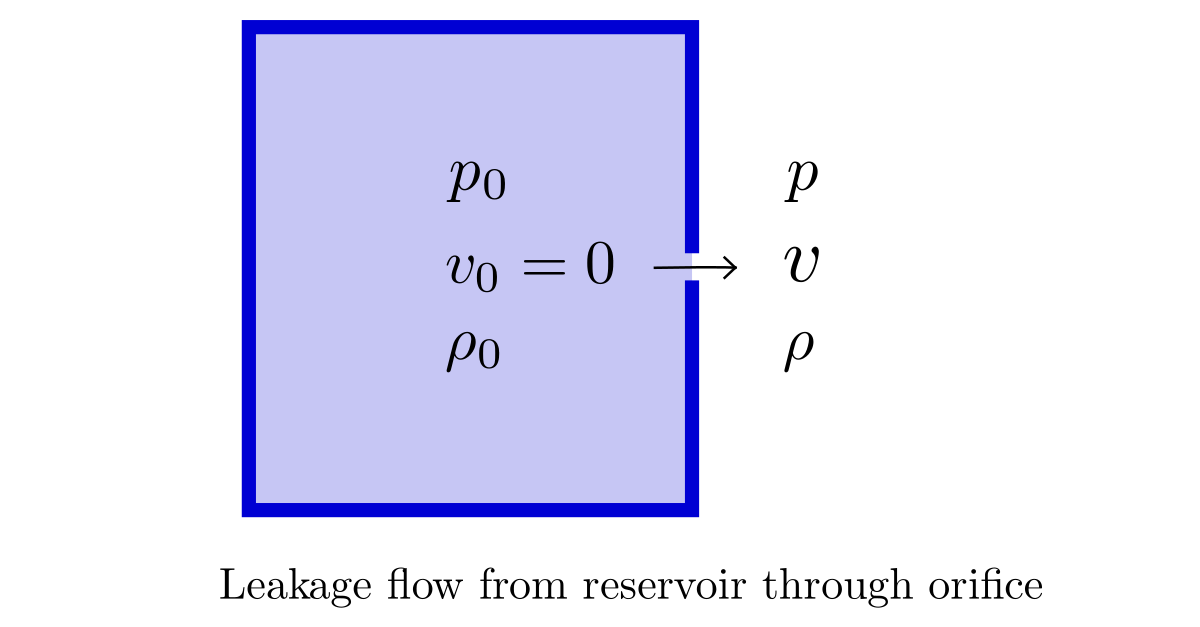
The velocity of fluid escaping from the reservoir through orifice is given by the following equation.

\[v = \sqrt{\left[2\left(\frac{\gamma}{\gamma-1}\right)\frac{p_{0}}{\rho_{0}}(1-r^{(\gamma-1)/\gamma})\right]}\]

where

\(v\) is the flow velocity through orifice
\(\gamma\) is the ratio of specific heats \(C_p/C_v\)
\(p_0\) is the pressure in reservoir`
\(\rho_0\) is the density in reservoir`
\(r=p_0/p\) is the pressure ratio across orifice`

The density inside the reservoir \(\rho_0\) can be obtained using the ideal gas law

\[\rho_0 = \frac{p_0}{R_gT_0}\]

where

\(R_g\) is the specific gas constant
\(T_0\) is the temperature in the reservoir in K`

The specific gas constant is obtained as

\[R_g = \frac{R_u}{MW}\]

where

\(R_u\) is the universal gas constant (=8314 J/kmol.K)
\(MW\) is the molecular mass of the fluid in kg/kmol or gm/mol`

The mass flow rate of the fluid escaping through the orifice can be obtained as follows:

(1)\[\dot{m}=Av\rho\]

where

\(\dot{m}\) is the leakage mass flow rate
\(A\) is the area of the orifice`
\(\rho\) is the density of fluid as it just escapes the orifice`

Using isentropic process relationships we have

(2)\[\rho = \rho_0r^{1/\gamma}\]

Substituting the value of \(v\) and \(\rho\) in (1) we get

\[\dot{m} = A\rho_0\sqrt{\left[2\left(\frac{\gamma}{\gamma-1}\right)\frac{p_{0}}{\rho_{0}}r^{2/\gamma}(1-r^{(\gamma-1)/\gamma})\right]}\]

In actual practice, the flow will be less than what is derived above and this is addressed by introducing the coefficient of discharge term \(C_d\). Introducing that in the above equation we get the final form of the equation which is also popular by the name Saint Venant Equation.

Important

Saint Venant Equation

\[\dot{m} = C_dA\rho_0\sqrt{\left[2\left(\frac{\gamma}{\gamma-1}\right)\frac{p_{0}}{\rho_{0}}r^{2/\gamma}(1-r^{(\gamma-1)/\gamma})\right]}\]